 GCSE Maths Quadratic functions transformation | Scale up Scale down
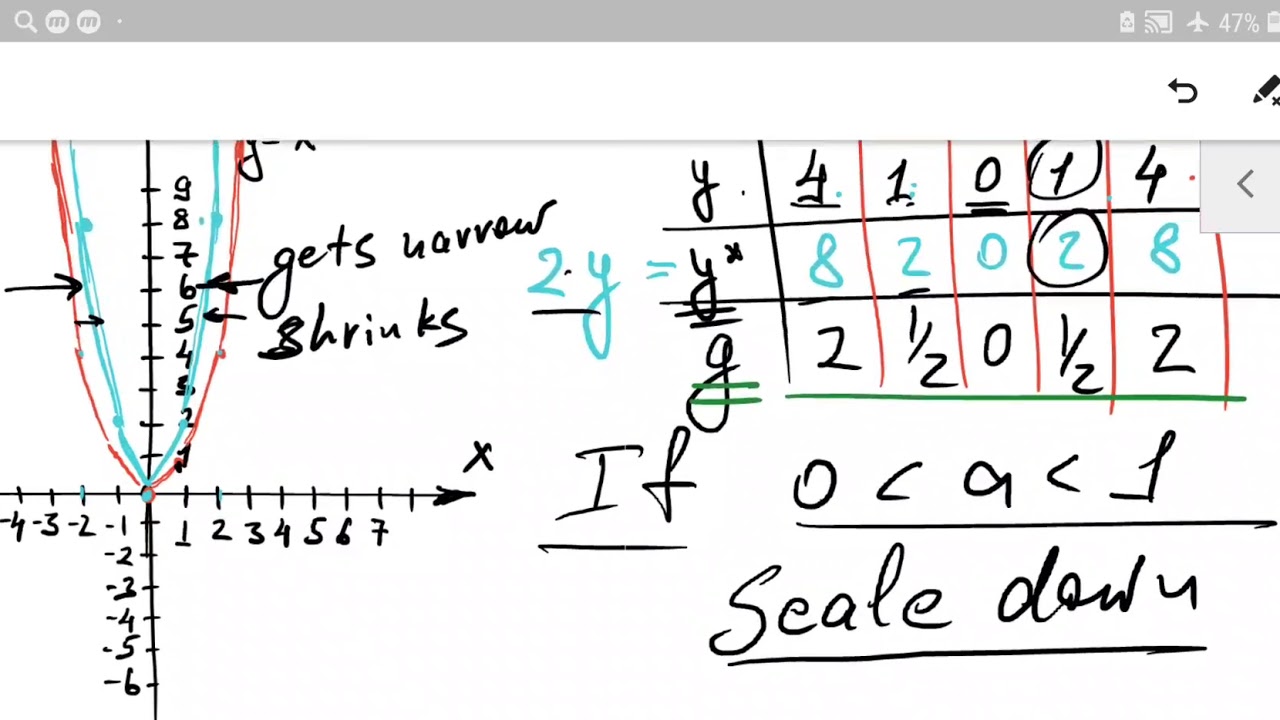
GCSE Maths Quadratic functions transformation | Scale up Scale down We continue exploring specific Quadratic Functions and today I will show you what in which way the leading coefficient a in the standard equation of the quadratic function y = ax^2 + bx + c might have an effect on the graph of the quadratic function. Yes, we will consider transformation " Scale up / Scale down " . However, visually it will be perceived as a horizontal stretch or compression (or shrink). Parabola gets narrower or wider depending on the value of the coefficient a in the term ax^2. You will see a visual effect for Quadratic Functions.
This topic refers to Quadratic equations and Quadratic functions from GCSE MATHs Algebra.
A bit of history:
The graphs of the quadratic function is called parabola. The parabola curve might be obtained by applying a cross-section to a solid " Cone ".
The cross-section should be applied in an inclined way, so that to arrange exactly parabolic curve. The Ancient Greek mathematicians were fascinated by conic sections. The name " parabola " comes from the Greek word for thrown because when any object is thrown, its path makes a parabolic arc. You can also have a reference to Physics | Mechanics (Kinematics to be precisely) you will find the curve that makes a projectile when thrown. There are many other examples of parabolas in now days: parabolic mirrors, radar discs, satellite aerials and radio telescopes because of their special geometric properties, focus of the parabola.
A quadratic equation is an equation of the form y = ax^2 + bx + c, where a, b and c are constants or coefficients. The graph of a quadratic equation is in the shape of a parabola which can either face up or face down.
To graph a quadratic function with a general equation y = ax^2 + bx + c, we need to know some essential parts of the function theory including the vertex for a parabola and the transformations that might take place. The vertex of a parabola is the turning point of the parabola. It is the point on the parabola at which the curve changes from increasing to decreasing or vice-versa. The transformation can be a vertical/horizontal shift, a stretch/compression or a reflection.
Given a quadratic equation in the vertex form i.e. y = a(x - h)^2 + k, the vertex of the parabola formed by the equation is given by (h, k). Knowing the vertex of the graph and the parent graph, we can then apply the required transformation to obtain the required graph.
In order to understand how to plot Graphs you just need to remember how to answer the question:
-FINDING y GIVEN x
Here is a reference to the video :
Parabola properties for the simplest quadratic function y=x^2:
1. The curve is a parabola and it opens upwards.
2. There are no negative y values, i.e., the curve does not go below the x-axis
3. The curve is symmetrical about the y-axis
4. The curve has a turning point or vertex at (0, 0)
This topic you will definitely meet in your further study on A-Level (AS Level) or on IB DP ( Quadratic expressions and equations and Quadratic functions)
I hope the video will enhance your confidence and give you a required practice
Don’t forget to suggest my channel to someone who needs it or looking for clear explanation :
-----Thank You for Watching-----
MATH SCIENCE
Yours, Daniel Dallas
For optimization
higher GCSE,GCSE higher tier, quadratic function transformation, qudratic functions scale, gcse maths quadratic transformation, GCSE MATHs quadratic equations best explaination, MATHs quadratic functions graphs,GCSE quadratic functions graphs,GCSE Quadratic functions best explained,qudratic functions clear explanation,parabola how to plot quadratic functions,how to plot parabola,GCSE MATHs parabola, gcse maths quadratic graphs, quadratic functions and transformations


0 Comments